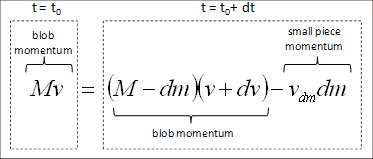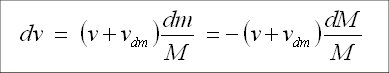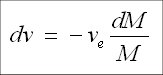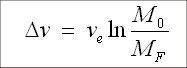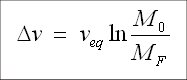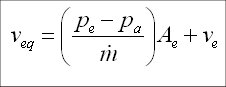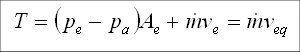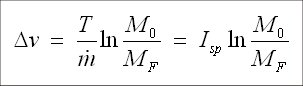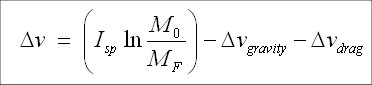Uncategorized – Page 4 – Liquid Rocket Engines (J-2X, RS-25, general)

J-2X Progress: Engine Assembly Complete

Just by chance, did you happen to see the title for this article? If not, please allow me the indulgence of repeating it…

Okay, I’m not ashamed. That felt good! We’ve all been working a long time to get to hoot and howl a bit about this. Wahoo! We are now officially into the next phase for J-2X development engine E10001.

Here, below on the left, was the engine sitting all cozy where it was assembled.

And on the right is the engine being lifted up and out of the assembly deck via an overhead crane. The techs then walked the engine out to the loading dock. There it was carefully loaded onto and mounted to a flatbed truck.

And next, our intrepid little will engine will brave the Mississippi heat on a gonzo road trip across the NASA Stennis Space Center to take up residence at the test stand. More on that coming soon!

J-2X Progress: Engine Assembly, Volume 5

The first car that I ever owned was a “goldenrod” 1974 Ford Pinto. My uncle Johnny was a car salesman for Buick at the time. He sold new, bright, and shiny machines in his showroom, but, of course, they took in trades of all shapes and sizes and conditions, many of which they would not dare put back on their own lot. My little Pinto was just such a vehicle. I bought it from the obscure and hidden back lot for $500 with absolutely, positively no guarantee that it would continue running until the end of the week. While it was only eight years old, back then (sad to say) autos simply didn’t last as long as they seem to today, particularly if driven hard in the weather of the northeast. So I spent months and months of afterschool hours and weekends replacing rusted panels and floorboards with broad swaths of fiberglass. Also, despite the fact that I was never a true gear-head, I was able to open up the hood and do some work on the engine. It was a straight four cylinders and as simple as could be. You could reach into the engine compartment and easily find and touch just about every greasy major component.

Just a couple of years later, my mother bought a new Saab. When I opened the hood of that sleek machine, I couldn’t find anything. The engine was turned sideways, apparently, and the compartment was completely stuffed to the gills with overly clean, boxed-up things that I couldn’t identify, and lines zigging and zagging in all directions. It was fuel-injected and front-wheel-drive and all kinds of other jet-engine craziness that my cozy little, oil-burning Pinto was not. So I closed the hood of that pretty blue Saab and I decided that I’d never make it as a modern mechanic.

Why am I bringing this up? Because some of the latest pictures from the assembly of J-2X Engine 10001 reminded me of my confusion in looking under the hood of my mother’s car. Only much worse. Yikes.

I’ll get to the progress we’ve made with the big pieces, but first I want to talk briefly about what looks like a chaotic explosion of confusing stuff all over the engine pictures. I will begin by telling you a secret: There are two reasons that we test rocket engines. First, we test rocket engines so as to impress our friends, most of whom are geek engineers like ourselves or people otherwise excited by loud noises made by neato, exotic machines (i.e., lots of NASCAR fans).

Second, we test rocket engines to gather data. Lots and lots of data. Many gigabytes of data. We measure pressures, temperatures, rotational speeds, flow rates, and dynamic vibrations both in terms of acceleration and in terms of strain. As part of the development program, we test in order to prove that what we thought was true when we created and analyzed the design is in fact true with the real hardware. And anywhere where we were mistaken, we need to know as quickly as possible so that we can recalculate our margins of safety and, if necessary, make adjustments to the design for the next development engine to be tested. Testing rocket engines is not cheap, and, of course, neither are the engines themselves, but we need to acquire this thorough, unassailable understanding of the engine long before we would ever imagine strapping a human life to something with such awesome power.

This need for data explains much of the “bloom” of seemingly ten thousand little lines and wires snaking hither and yon all over the engine. Some of these small lines carry pneumatic pressure for actuating valves or providing purges, but lots of them are related to the many, many measurements we’ll be taking during the upcoming hot-fire test series. In fact, these early development engines will be far more heavily instrumented than any engine we would ever fly because, again, their whole purpose to generate useful data (well, that and to impress our friends). What is truly amazing to realize is the fact that every one of these lines has a specific shape and route; every one of these lines is designed, not randomly configured; and every one of these lines represents a documented step in the assembly process.

Now to the big stuff.

The first big assembly news to share was the arrival of the regeneratively-cooled portion of the nozzle (usually called the “regen nozzle” around here). In the pictures above, on the left you can see the assembly technicians preparing to lift the regen nozzle out of its shipping container. On the right is a picture of some preparations being made to the nozzle before the rest of the engine is stacked on top. If you’ll remember, the rest of the engine was previously being assembled on a simulator of the nozzle. Interesting little side note: In order for the technician to get into the position you see in the picture on the right, she had to crawl under the elevated assembly platform and stand up inside the nozzle. In the picture below you can see the stack of the engine on the actual regen nozzle.

Now, with the regen nozzle in place, lots more stuff could be added to the assembly. In the collage below, five big items are shown in the process of installation: (1) the liquid oxygen pump discharge duct that carries liquid oxygen from the pump to the main oxidizer valve, (2) the oxidizer turbine discharge duct that contains within it the helium heat exchanger, (3) the turbine cross-over duct that carries hot turbine drive gas from the hydrogen turbopump turbine outlet to the oxidizer turbopump turbine inlet, (4) the main fuel valve, and (5) the fuel pump discharge duct that carries liquid hydrogen from the fuel pump to the main fuel valve.

And that’s just a small sampling of the flurry of assembly activity over the last few weeks. I like the fact that I was able to include pictures showing some of the folks working hard to get this thing put together. They’re doing an extraordinary job. The result of all this work is something that is really, truly starting to look like a rocket engine. The pictures below show what you’d see if you took a casual stroll around the engine. Note that the regen nozzle is covered with a black, protective tarp and there are other protective coverings all over the engine, so this isn’t quite as gorgeous as it could be, but for us rocket geeks it’s awfully compelling.

So, that’s where the engine stands. Almost all of the big stuff is installed, with just a couple of notable exceptions. Additionally, lots and lots of little stuff has also been installed. Over the past few weeks, it’s come a long, long way. As a reminder, take a look at this:

But, then, I guess everything eventually comes a long way with a little bit of hard work. Take, for example, how I’ve been blessed to evolve through the years:

With all due respect to the Ford Motor Company and their venerable line of Pinto automobiles, that’s one heck of a leap even if it did take a long time. I just bet that my Uncle Johnny would be proud to think that he was there at the start of the whole thing.

J-2X Extra Rocket Alchemy: How Paper Becomes Precious Metal

Alchemy isn’t practiced much these days in the medieval sense. That’s a shame. A little extra gold would be useful. But there is little doubt in my mind that alchemy is exactly what is going on behind the scenes, deep within the dungeons of NASA as we develop a rocket engine.

How do I know? Because I have been one of the chief alchemists for J-2X (…in fact, I kind of like that title: “Manager of Alchemy”). No, we don’t start with a heap of scrap metal and end up with bars of gold or the philosopher’s stone. Rather, we start with a heap of paper and end up with a rocket engine – and, well, a larger heap of paper. As much as bending and grinding and welding and balancing and casting and torquing, the story of the paperwork is big part of how a rocket engine comes to be.

Hold on! Yes, I am endeavoring to present here a blog article about paperwork and, further, I am endeavoring to make it (reasonably) interesting. Really. All along I have tried to convey the complexity and scope of what it takes to design and develop a rocket engine. It ain’t easy. That’s why not everyone does it and even we don’t do it very often. And, like it or not, paperwork is what makes it all happen.

Here is how we at NASA develop launch systems:

Joe Mega-genius wakes up with a vision for what NASA ought to be doing. He alone has the image in his mind for a launch system for exploring space. He single-handedly directs everyone at NASA and every contractor as to what to do, what analyses to run, how thick the metal of a feedline needs to be, how the launch pad should be configured, the shapes of the knobs on astronaut’s control panels, the material to be used for the springs in the check valves on the engine, everything. He personally takes care of all management and business and procurement activities so that there is a single, non-contradictory point of contact for everything. And nobody at any other NASA center or Congress or the Administration questions what he says so he gets all the money in the world to spend as he sees fit because, after all, he is Joe Mega-genius.

All it takes is one brilliant focal point and everything falls into place easily.

Guess what: That’s a lie. This is not how it works. Further, that is NEVER how it worked. Never. All cinematic or mythological approximations of that scenario are, simply, myths. Nobody can know that much or do that much. It takes many, many people and (good) paperwork is the key to get many, many people successfully coordinated.

Note that specifically what I want to talk about is the paperwork that enables and facilitates development. That is somewhat different than the many products that result from a development effort other than the hardware itself. Perhaps we can talk about all of those other products at another time. I also don’t want to talk about the paperwork at the highest levels, between the Federal Administration and Congress, the Office of Management and Budget (OMB) and agency authorization bills and appropriations bills, etc. Interesting stuff, but not quite right for this blog (to say the least).

Let’s start with the assumption that we have a mission. Let’s say that it is simply this: Get XX payload to low earth orbit. Okay, simple enough.

How? Well, there are lots of ways to launch something. There are different rocket configurations using different propellants in different combinations. Each potential solution brings with it certain positive and negative aspects, consequences far and wide. So, we have a trade study and come up with a “best” solution. That “best” solution has a particular configuration and piece parts that all have to work together. So, we write down what those various parts all have to do. Those are the top-level requirements.

So, we start with one mission objective and now we have requirements for the launch vehicle itself, for the launch pad, and for the launch control center. If we just focus on the launch vehicle, we then have to further break down the requirements into separate groups for the payload section – including, of course, a crew element if we’re putting people in space – and the various stages and boosters. And, on those stages there will be engines, so you have to break down the stage requirements into separate groupings for the engines and the tanks and propellant feed systems and the structures and the electrical systems and guidance and control systems.

Thus, you start with one extremely broad mission and, in just a few steps, have dozens of separate groupings of requirements for dozens of different things that all need to work together. At each step along the way, you need to figure out a conceptual design, i.e., a series of plausible configurations and functions for the various components, and then assign those necessary characteristics as requirements before you take the next step downwards. And if you should decide at any point along the way that you want to change something higher up in the chain, then, boom, you have repercussions throughout everything below.

This whole process is called requirements decomposition. The management of the process represents a huge task at the early part of a development program and remains important throughout as you head into the verification phase where you demonstrate that every requirement has been fulfilled. In the diagram below, you see the decomposition process. Every place where there is a dashed line and a red dot I’ve loft out details, i.e., lots more stuff.

What is the result of all this by the time it gets to, say, the J-2X? Well, for J-2X we’ve got just about 200 requirements dictated to our development effort from the vehicle project level. These requirements cover performance characteristics and physical properties and functionality and safety and operations considerations and standards as to how things are made and analyzed, plus a bevy of items dedicated to how the engine effectively interacts with the stage. These requirements define, at the top level, what the J-2X shall be when development is complete.

Now what? You’re at the beginning stages of a development effort and you’ve got in hand a book of requirements to fulfill, so what do you do next? You make plans.
• You create a plan for the overall development effort. This includes how many development engines are you going to build and test, how many tests you’re going to run, where you’re going to run those tests, what you’re going to do in terms of testing at the component level, what analyses are you going to do, what level of non-destructive and destructive evaluation will you be performing on built and/or tested hardware.
• You also make plans for how you’re going to manage the office and manage the various “office disciplines” such as risk management, business management, systems engineering and management, and configuration management.
• You have an overall plan for how and what safety-related assessments and analyses are going to be done and by when. This needs to be tightly coordinated with other elements of the launch architecture since the whole notion of safety is a wholly integrated consideration.
• You have to generate a clear plan and process for how decisions are going to be made since nearly all big decisions have budgetary or schedule or technical risk implications. To enable these processes you issue charters to delegate formal authority for decision-making boards.
• And there’s much more…

So, that’s how you get started. In the beginning, you don’t usually have any hardware. All you have is paper (or, these days, computer files). And you’ll hear lots of people complain about “paperwork this and paperwork that,” but without this foundation of documentation, nothing else can follow. What does come next beyond this foundation is that you fulfill your plans. You do the design, do the analyses, create the drawings, fabricate the hardware, assemble the engine(s), and then test the hardware to demonstrate that you’ve met the imposed requirements.

Thus, just as the rocket engine is itself a complex machine, so too is the infrastructure coordinating the efforts of hundreds of people to arrive at the final product. In some ways, it’s even more complex than the rocket itself: imagine a shiny rocket engine rising out of a pile of paperwork. It is truly, in the end, almost alchemy.

J-2X Progress: Engine Assembly, Volume 4

It’s been about three weeks since I last reported on the progress of J-2X Engine 10001 assembly. What I would like to do is show you pictures of great big additions to the engine, but the truth is that for the last few weeks the engineers and technicians have been diligently plugging away at smaller stuff, at the details.

“It’s the little details that are vital. Little things make big things happen.” – John Wooden

I would hazard to guess that Coach Wooden was talking about basketball – or maybe life in general – when he spoke these words, but on a rocket engine, everything has to work so the details of the big stuff and of the little stuff are equally vital.

So, here are some pictures and some details…

We’ll start with a picture of the overall assembly and the addition of the item in the dashed, red oval on the right-hand side of the picture. That is the gas generator. Yep, that little thing powers both turbopumps and therefore is the driving force behind the whole engine. Here is a closer picture.

As I’ve mentioned before, during the whole assembly process, things either have covers attached or are wrapped in tape and other protective media so that no debris gets in the engine and so that delicate parts are not damaged. You can see that here on the gas generator.

Note that in the image of the gas generator above, you will see the “U-duct” from an earlier blog series entry regarding Direct Metal Laser Sintering. This, right here on the engine, is the piece for which we may have found a future innovative manufacturing method.

Next is a picture of a mounting plate. This is not even a primary piece of the engine if you were looking at a schematic, but it’s a vital piece nonetheless. On this mounting panel sits the two Main Injector Exciter Units (MIEUs). An MIEU is analogous to the ignition coil in your automobile engine but with a good bit more punch. We use two units for redundancy to further ensure overall reliability. If, in the morning, your ignition coil goes dead in your station wagon, well you’re just stuck in your driveway. However, if during a launch mission you can’t get the J-2X started, then you’re stuck somewhere over the Atlantic Ocean at two or three hundred thousand feet. That’s a somewhat more treacherous situation. So we use very highly reliable parts and we use two parallel units.

What makes this mounting plate interesting is that it has vibration isolators so that the noise and shaking of the engine don’t impact the electrical components of the units. Those eight circles on the mounting plate are the tops of the isolators so there are four for each unit. Kinda cool little details.

Next, more mounts. In the picture below, you will see two rectangular items, each with eight holes. These are mounts on which will eventually reside pressure transducers. There are several such transducer mounts across the engine. These particular mounts are installed on the arms that hold the oxidizer turbopump. So, we have mounts installed on mounts and you can begin to understand the complex layering that an engine assembly represents.

One strategy that is used quite often during the assembly process is to do stuff unattached from the main assembly itself. Over on a separate bench, you put together a bunch of smaller pieces and then take this whole subassembly over to the engine for installation. Over the past couple of weeks, this is exactly what has been done with the ancillary line “raceway.” Below is the completed raceway subassembly.

All of the lines and brackets and nuts and bolts were separate pieces when the engineers and technicians began.

Each line in that assembly is different since each line represents different fluids coming to or coming from the engine at different pressures and temperatures. There is helium, oxygen, hydrogen, and even nitrogen. There are pressures over 3,000 psi and less than 50 psi. There are temperatures approaching the boiling point of water and those less than 400 degrees below zero Fahrenheit. Thus, some of these lines shrink from the cold. Some of them stiffen with high pressure. Yet all of them have to be flexible to accommodate the gimballing of the engine and every one of them is vital either to the engine or to the vehicle. In other words, it is a complex design, a complex manufacturing process, and a complex subassembly to put together. And it all has to be correct. Details, details, details!

Lastly, I’d like to toss in a cool picture from the test stand. Below is a picture from test stand A-2, with the engine mass simulator installed. This is where J-2X Engine 10001 will be tested. The brightly painted, yellow hunk of metal is supposed to weigh the same as a J-2X and have the same dimensions. It’s used for checkouts of various systems including the test stand.

Another thing of note in that picture from A-2 is large gray object to the right. That is half of a water-spray system that will be used during testing when no nozzle extension is installed on the engine. Near the top of the picture is a hinge painted bright red. When deployed, the gray water-spray structure will have been swung down so that its two pieces (the one shown and the other one to the left and out of the picture) join at the bottom of the engine nozzle. This is all part of the system to deal with the substantial, combustible, fast moving, and very hot exhaust from the engine during test. This water-spray system was installed specifically for J-2X testing.

That’s where we stand. The details of the engine assembly are coming together, with more parts arriving at the NASA Stennis Space Center every day, and with the test stand is being readied to accept installation of the engine. This is Volume 4 of the assembly saga. By the time Volume 5 is ready for the blog, we should be very close to having a fully assembled engine. Wahoo!

J-2X Extra: Supplier Appreciation

Pssssssst. Come over here. Yes, you. Come on. Right here. Lean in real close because I’ve got a secret to share. A little closer. Are you ready? Okay, here it is: rocket engines are complex machines with lots and lots of pieces.

Well, maybe that’s not much of a secret. Maybe that’s just about as much of a secret as, say, “water is wet.” But what might not be known too well is how many different people get involved in developing and building a new rocket engine. Sure, the NASA office is located here at the Marshall Space Flight Center in northern Alabama, and the facility of our prime contractor for J-2X, Pratt & Whitney Rocketdyne is located in Los Angeles, California, and our engine assembly and test facility is at the NASA Stennis Space Center is southern Mississippi, but we engage more of the country than just those three key locations. The J-2X development effort has 362 different suppliers and vendors in 35 states and 4 in other countries.

Now, we here on the NASA side don’t chose the suppliers for this project. We sometimes get involved in okaying a supplier for various reasons pertaining to regulations (blah, blah, blah…snore…zzzzzzz…for a really good time, sit down and read the Federal Acquisition Regulation!), but it is primarily the job of our prime contractor to figure out what is needed and to hire appropriately. It’s just like having a general contractor if you were building a house. They have connections and know who best to call for the plumbing or the roof or the tiling in the bathroom.

So, we don’t pick ’em, and we certainly don’t endorse anyone over anyone else, but when a company steps forward and does a whiz-bang job for us – and therefore for our space exploration mission – I think that they deserve “atta-boy” recognition. Much earlier in this blog series, I included a picture taken at the facility of Cain Tubular Products in St. Charles, Illinois. They are a relatively small company that supplies our heat exchanger coils and they’ve done a whiz-bang job for us. We have other suppliers that are Fortune 100, multinational corporations like, for example, Honeywell International that provides the J-2X engine controller and several of the valve actuators. They too generally do a whiz-bang job for us.

So, here I’m going to shout out an “atta-boy” to another supplier…

Omni Electo Motive Inc. is located in Newfield, New York just outside Ithaca (beautiful country up there). To give you a general idea of what they do, I will quote their website: “Omni Electro Motive Inc. is one of the world’s premier independent manufacturers of custom manufactured gas turbine blades and vanes for jet engines and gas turbine industries.”

Well, okay, but it is not only jet engines and gas turbines that have turbine blades, so do rocket engines. As I’ve discussed before, the power of the engine comes from the power of the turbopumps. The turbine blades are small airfoils that convert the power of flowing hot, high pressure, high velocity gases into rotational power. Thus, they are a key component of the engine. Between the two turbopumps, the J-2X has over 300 turbine blades. Below is a picture of some turbine blades prior to assembly into the J-2X fuel turbopump. Each blade is fits snugly like a glove into a disk connected to the rotating shaft of the turbopump so that only the airfoil section is exposed.

These turbine blades not very big (easily fit in the palm of your hand), but they need to be exceedingly well made. They see extreme environments and undergo extreme loads during engine operation. In essence, they need to be as flawless as the finest jewels. That is why it takes a specialized supplier like Omni to do the job. However, more than just providing excellent products, Omni has engaged with the J-2X development team on the design side through multiple design iterations. The application of their extensive experience in this specialized field has been positively vital to our success.

Below is a picture of Omni Electromotive Inc. President, Frank Deridder (on the right), receiving a Supplier Appreciation Award from the Pratt & Whitney Rocketdyne J-2X Program Office.

Thank you very much guys for your dedication and for your commitment to excellence. “Atta-boy” and keep up the good work!

Front Row, From Left to Right: Holli Maneval, Adam Kellerson, Donald Koski, Mark Clauson.

Back Row From Left to Right: Ray Hornbrook, Matthew Oelkers, Brian Card, Jamie Brooks, John Case, Steven Vallimont

J-2X Progress: Engine Assembly, Volume 3

Assembly of J-2X development Engine 10001 “officially” commenced on Monday 21 February of this year, just about a month ago. In even more important news, my own household had an eight-pound, eleven-ounce new arrival on Friday 11 February. No, no, not that kind of arrival. Rather, it’s a new puppy named Kate (…had ya fooled for a moment, didn’t I?).

Thus, I have the great pleasure of spending this spring watching both J-2X Engine 10001 and Kate grow up and into what they will finally become. Here is a picture of Kate cuddled with our cat Cassady to give you an idea of her size when first she came home. By the way, the cats all thought that Kate was just swell so long as she was unconscious and inert. Their opinion changed significantly when she woke up.

And here was the “engine” as a pup, newly weaned and delivered to Stennis Space Center. It began as just the Main Injector stacked on the Main Combustion Chamber. Humble beginnings.

Here is the engine today. Awwwww, our little baby is growing up so fast!

The most noticeable additions since the last time that we checked in on the assembly progress are the two inlet ducts stacked on top of the two turbopumps. More stuff has been going on, like the attachment of brackets and mounts for parts to follow, but those two ducts are the biggest items. So, let’s talk about them…

In the picture of the stacked engine assembly, the inlet ducts are encased in protective coverings. In the picture above, however, these coverings have been largely removed from the fuel-side duct for inspection purposes. It looks kinda funky, huh? Well, despite the oft-demonstrated good fashion sense and excellent taste of your average rocket scientist, we don’t usually make thinks look funky just so they look cool. There is usually a function to our funky-ness.

Here is the function: The engine has to gimbal. What that means is that you have to be able to point the engine in slightly other directions besides straight down from the back of the vehicle stage. You can think of gimballing as being like a rudder on the back of a boat but in three-dimensions. Thus, the whole engine has to pivot around when pushed or pulled by two large hydraulic actuator arms from the stage.

In order to accommodate this movement of the engine, the inlet ducts need to be able to bend and flex and even twist a little bit. So, in order to make super-duper-strong metal ducts flexible, you have to add the convolutions like you see above. It’s very much like one of those twisty drinking straws that I absolutely loved as a kid (see, even then I was unconsciously thinking about rocket engine components). If you bend a regular drinking straw, the thing collapses in on itself. If you bend one of those twisty drinking straws with the convolutions, the straw remains useful as a duct. The external structures in the picture of the inlet ducts, those shiny triangular brackets hinged together, hold the whole thing together under operational conditions.

Here’s a neato little fact about the fuel-side inlet duct. In order for the convolutions to do their job by allowing movement, you can’t have any ice buildup in the convolutions. Liquid hydrogen is so cold that it can freeze nitrogen right out of the air. If you don’t have some way to insulate this duct, it would be covered in nitrogen ice while sitting in compartment at the back of the stage. But you can’t insulate it on the outside or, again, the thing can’t flex. So, what we’ve done is make the whole thing into a Thermos® bottle. There are actually two walls of convolutions, an inner wall and an outer wall. In between is a vacuum and that vacuum provides the insulation. This feature is not necessary on the oxidizer side since liquid oxygen will not freeze anything out of the air other than moisture. Thus, so long as you keep the compartment free of humidity, the oxidizer side is fine with only one layer and bare metal (though special accommodations are necessary on the test stand where the ambient environment cannot be so carefully controlled).

So, that’s the status of the J-2X Engine 10001 assembly and a description of its inlet ducts. The status of Kate is that she is growing like a weed. Every day her legs look longer. Of course, it was only after we adopted her that the shelter people seriously and openly speculated that the father might, in fact, have been a Rottweiler. Oh dear. She’s nearly 24 pounds at less than fourteen weeks old. The cats are more displeased with each passing day.

J-2X Doghouse: The Rocket Equation! Wahoo!

Welcome back to the J-2X Doghouse. We’ve talked before about what a rocket actually is and we’ve talked about different kinds of rocket engine cycles and where J-2X fits in that family. This time, in response to a couple of early requests on this blog, I’d like to talk about rocket engine performance characteristics and how they relate to successfully getting off the planet and into orbit. Because this comes down to a matter of equations – and therefore at least half of the reading audience will click on their Facebook icon as soon as they see any equations – let me start with an old joke:

Q: What do engineers use for birth control?
A: Their personalities.

Of course you know that joke can’t be entirely true if you’ve read any of the articles profiling the J-2X office. Just about everyone in the office have children. But somewhere, deep down, in order for that joke to have lasted so long, there must be a tiny sliver of truth. Okay, yes, I admit it, here it is: People who become engineers do so for a whole variety of reasons but typically share in common an aptitude towards mathematics and a desire to know how things work.

For me, this whole “future-engineer” notion translated to an appreciation of physics and the representation of the real world, to some approximation, in equations. Seriously, just think about that for a moment. You can pick up a pencil, draw a simple sketch, apply some fundamental laws, and, boom, you’ve got a prediction right there on your paper for how the real world will function. Now that’s darn exciting! At least it is to me. But, okay, word of advice: Showing this level of enthusiasm regarding physics and neato equations is generally NOT good fodder for a first date. Trust me.

However, since I’ve not had a “first date” in over a quarter of a century, I am now going to explain the derivation of the foundational equation for all rocketry: The Rocket Equation. Approximately 99.7% of the world’s population at large does not know this … and, yes, that is a 100% unverified, made-up statistic. Regardless, today you will join an elite, exclusive, and fashionably eccentric club.

[Warning: Some of the mathematics gets a bit heavy here, but some of y’all asked for it.]

First, we start with a simple drawing. Please note that my wife is the artist in the family; I “draw” in PowerPoint. Sorry.

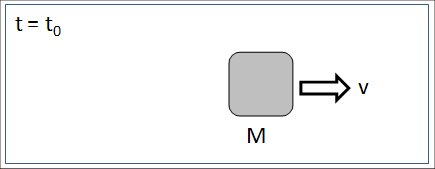
What you have is a thing, a blob, at time equal to t0 with a mass of M moving at a velocity of v. At this point, don’t think of the blob as a rocket. It’s just a thing in an imaginary space where there is no gravity, no friction, no environmental impacts at all.

We then go to the next step in time, time = t0 + dt, where dt is a small increment.

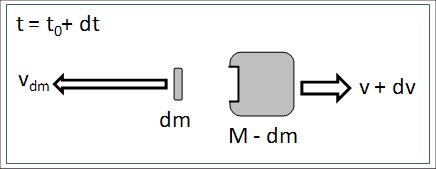
Our blob has ejected from itself a small piece of mass, dm, in the opposite direction from which it was moving. The small mass has a velocity of vdm in the opposite direction of the original blob. The blob, by the way, has a mass now diminished by dm and a velocity that has changed by some increment dv.

Do you want to play the gray blob at home? Okay, do this. On a smooth floor, tile perhaps, sit in a rolling chair holding a basketball. Throw the basketball. You, still in the chair, will roll in the opposite direction from the flight of the basketball. Your initial velocity, v, was zero. Your initial mass, M, included you, the chair, and the basketball. Your new velocity is zero + dv. Your new mass is now minus that of the basketball, dm, flying in the other direction. Ta-da!

Now, how do we turn this simple concept and simplistic drawings into rocket science? Simple, we call up the work of our friend Sir Issac Newton (1642 – 1727). Newton’s First Law of Motion states: “An object at rest tends to stay at rest and that an object in uniform motion tends to stay in uniform motion unless acted upon by a net external force.” I told you that there were no external forces acting on our blob and I drew the box around the whole thing, blob and small piece together. Combining the philosophy of this First Law with the mathematics of the Second Law results in a simple conclusion that in absence of any external forces, momentum is conserved. So, the total momentum in the first drawing is the same as the total momentum in the second drawing with momentum defined as mass × velocity.

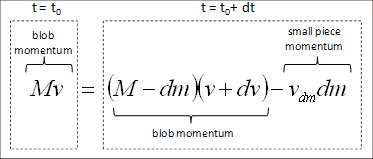
The second term in the right-hand box is negative since the velocity of the small piece is in the opposite direction as the original movement of the blob. Okay, now multiply this all out and eliminate redundant terms and – thanks to some niceties of differential calculus – eliminate second order terms to yield the following:

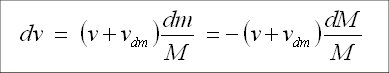
The dm = -dM switch-a-roo is possible since the incremental change in mass of the blob over the time period dt is exactly the negative of the piece ejected.

Rather than talking in terms of absolute velocity of the blob and the small piece, let’s instead talk in terms of ve, the “ejection velocity” of the small piece. So, this is a relative speed. Then the equation becomes:

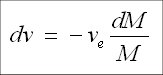
Believe it or not, that’s it. In its most rudimentary, simplified form, that the Rocket Equation expressed over a very small time increment dt. If you add up a bunch of these very small time increments, or in other words integrate over a measureable time period [Oh no, integral calculus buried in a blog! Call the blog police!], you get the following:

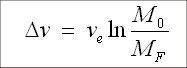
What does this say? Equations always say something or they’re worthless. It says that the change in velocity of a blob is equal to the relative ejection velocity of small pieces flung away from the blob times the natural log of the ratio of initial mass, M0, to final mass of the blob MF. The natural log thing got in there thanks to the rules of integral calculus. You’ll have to trust me on that one.

Now, what does this have to do with rockets? Well, how about rather than ejecting small discrete chunks of mass we think about what a rocket engine does, which is spew out a continuous stream of mass in the form of high-speed hot gases. The whole derivation above holds for that case with one modification. The hot gases ejecting from the nozzle create a pressure field at the point of ejection. That pressure field creates a force acting on the system. Because there is a force involved now, momentum is not conserved. The derivation is a bit more complicated, but it’s not too bad. The result looks like this:

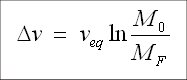
Where ueq is called the “equivalent exhaust velocity” and is defined as:

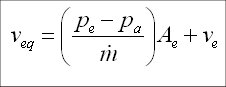
The first portion of that last equation deals with the pressure field. Basically, it is the exhaust pressure at the end of the nozzle, Pe, minus the ambient pressure outside, Pa, times the exit area of the nozzle, Ae. Force equals pressure acting over an area. Simple. The “m-dot” term is the mass flow of the hot gases out of the nozzle.

We’re almost there. Really. Hold on.

Next, I want to define thrust. We could have started this way by drawing a control volume around a rocket, but I like starting with the blob. Thrust, T, is the force that the engine imparts on the vehicle. So, it includes the pressure field aspect and it includes the aspect of ejecting hot gases at high speeds. Here it is:

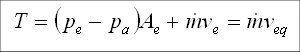
If you put a rocket engine on the test stand, fire it, and measure how hard it pushes against the stand, this is what you are measuring. Sometimes you will see a rocket engine specification that will talk about “vacuum thrust” or “sea level thrust.” The difference between those can be found in the ambient pressure term, Pa, in the equation above. In a vacuum, Pa = 0. At sea level, Pa = 14.7 pounds-force per square inch. Note that depending on your system of measurements, there could be a “g-factor” conversion lurking in the mass flux term so be careful.

Substituting back into the Rocket Equation, we get this:

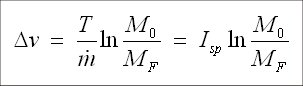
I have now cleverly introduced the concept of specific impulse, Isp, which is thrust divided by mass flowrate. When talking about rocket engines, we typically describe this parameter as being analogous to gas mileage so that people can understand, but here you can see that it’s an integral part of the basic physics of the acceleration of a rocket vehicle. (Again, beware of hidden g-factor conversions.)

Note that earlier I said that we didn’t have any gravity in our hypothetical situation and that we didn’t have any friction. I can now add these things into our system a simplistic manner to facilitate the final discussion and to present the final equation:

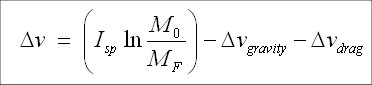
That, right there, believe it or not, tells you 90% of the whole story about how rockets get into orbit and even how they go from there into the rest of the cosmos. What do you need to get into and stay in orbit? A lot of velocity. And this equation tells you all about it. Listen carefully to rocket scientists talking about “delta-v” in movies or the news or in documentaries. “Delta-v” is everything. You need so much delta-v to get to orbit. You need so much delta-v to change orbits. You need so much delta-v to get out of orbit and head towards the moon or anywhere else. Whenever you hear this, they are referring to the Rocket Equation.

Let’s break it down by starting with the last two terms on the right-hand side. These are loss terms and that’s why they are negative. First, as long as you are gaining altitude, you are fighting against gravity. If we had some way to dial down gravity, we could launch rockets more efficiently because this term would lessen (we’d also all float away). That’s intuitive. It takes energy to lift something. Second, as long as you have friction caused by drag against the atmosphere, you’ve got losses. Compared to a vacuum, especially at high speeds, our atmosphere is like soup to a launch vehicle and you need energy to overcome it. Thus, both of these loss terms tell you that for greatest efficiency, it’s best to get up high, out of the atmosphere, and level out to stop fighting gravity as fast as you possibly can. And that’s exactly how we launch rockets. It’s not an accident or a whim. It’s physics.

Now, the first term on the right-hand side in that final equation has two pieces. First, there is specific impulse, Isp. That is a measurement of how efficiently the rocket engine produces thrust. For a given amount of propellant the engine produces this much thrust. Second, there is the ratio of masses within the natural logarithm. What this says is that the lower the final mass of the vehicle is relative to the starting mass, the more velocity can be gained. So, what you want is very low final, burn-out mass as compared to where you started. When you get to the end, you don’t want much by way of leftover, unused propellants, and you want as little superfluous structure as possible. Remember, part of your final mass is your payload, which is the satellite or your capsule filled with astronauts. That’s the important stuff. It’s this notion of discarding unnecessary and heavy stuff along the way that results in the reason why rockets typically have multiple stages. As you go along, you toss off heavy structures that you no longer need: The more stuff that you can shed, the less that you have to carry along, and the more velocity that you can pick up. Again, it’s intuitive.

If you’ve made it this far and if you’ve grasped the basic concepts of the physics involved, you truly know more about rocketry than almost anybody you’ll meet. And it’s amazing how intuitive it all ends up once you’ve plowed through the mathematics. Good equations are those that can tell a good story. The Rocket Equation is one such equation.

My recommendation is that you print this out and take it along with you on your next date. Really, you’ll be a big hit! (Or not.)

J-2X Progress: Engine Assembly Continues

Once upon a time, I used to consider myself reasonably handy with a saw and a drill and a miter box and various rudimentary woodworking tools. I certainly knew my limits, so I never did anything too complex, but most of the fun from pursuing such projects was the creativity involved. I didn’t plan out a great deal. I preferred an evolving, organic (i.e., lazy) approach. Given the nature of the forgiving materials involved, that was generally fine. In my wife’s art studio, there’s a cat tree with six or seven beds that fills an entire wall. I built it with no drawings, kind of on the fly, and it still turned out okay (or, at least, the cats seem to think so).

That is not, however, how you assemble a rocket engine. You don’t wing it. You plan everything. The materials are not forgiving. Just about everything is heavy and, if you drop it, or scratch it, or scuff it, or nick it, then you have the joyful experience of traipsing through a paperwork exercise to make sure that whatever you damaged is still usable. In other words, rocket engine pieces are both extremely rugged and rigid yet also precisely machined and fragile. Oh, and unlike two-by-fours, rocket engine parts aren’t cheap and as easily replaced as a trip down the street to Home Depot. Thus, a great deal of time spent poring through the planning documentation and lifting and moving things with exceptional care.

You can think of a rocket engine as a large, three-dimensional jigsaw puzzle. The pieces have to fit together exactly and properly. To make this happen, you first have to have really well-manufactured parts, but then you also need good ground support equipment (GSE) and knowledgeable, competent, and dedicated technicians. Below is a picture of one critical piece of GSE, the engine build dolly. This is essentially a rolling piece of elevated floor onto which you build in the engine. Considering with the engine sitting on it, plus tooling, plus the technicians themselves doing the work that the dolly could be holding well over 6,000 pounds, this is a stout piece of equipment.

When I built the big cat tree, I just moved around the mess in my garage and I pulled my pickup out of the driveway to lay out the bigger pieces. The picture below is the J-2X assembly area. Note that there aren’t any flower pots with last year’s dead petunias, or half-empty bags of bird seed, or cast-off, half-used rolls of duct tape scattered about the floor. In other words, it doesn’t look like my garage. It is extremely clean and orderly. It is a FOD-free zone: FOD = foreign object debris. When assembling an engine, you do not want ANYTHING in the engine that does not belong there. I will be showing you pictures below of various stages of engine assembly so far and you will notice that there is tape and/or plastic closures over every open hole where something might accidentally fall. One dropped nut or hunk of wire or wad of tape and you’re either forced into a costly disassembly exercise to get the stuff out or, worst case should the FOD be missed and left in the engine, you could have an engine failure in test and the loss of tens of millions of dollars of hardware.

The other thing that you will notice from the picture of the assembly area is how well the whole thing fits together. Pieces of the floor retract to allow for the dolly to be positioned in the middle. The kit carts have bays into which they can slide for convenient access to the necessary hardware. There is an overhead boom with commodities available for when the assembly and checkout processes require gasses or electrical power or a hookup to a simulated vehicle stage computer. And, of course, just above the boundary of the picture is an overhead crane for lifting operations.

Now, can you just imagine the magnitude and glory of my cat tree if my garage was so neat, well organized, and fully equipped? Difficult to fathom, huh?

So, where does the engine assembly stand? Since I last reported the initiation of assembly, great strides have been made. Let’s step through the biggest pieces of the sequence. First, the birdcage was put into place on the build dolly. Remember, the yellow birdcage is a simulator for the first portion of the nozzle. Later, it will be replaced with the real nozzle. The dolly was then wheeled into place in the assembly area.

Below is the next sequence. The picture farthest on the left is the MCE, i.e., the main combustion element (composed of the main injector attached to the main combustion chamber) sitting in its shipping box. In the middle is a picture of the MCE with the turbopump arms installed. From these four heavy arms will be hung the fuel turbopump and the oxidizer turbopump. And, on the right, is a picture of this the whole assembly of MCE with the turbopump arms mounted on top of the birdcage.

Next, the two turbopumps were installed, first the oxidizer turbopump and then the fuel turbopump. I can state that here quite simply in a single sentence, but go back to that series of pictures above: planning, lifting, moving, positioning, etc. A great deal of careful work went into each step.

Now, I don’t know about you, but this is getting darn exciting for me. If I squint hard at that last picture and add some ducts in my mind, then that really looks a whole lot like an honest to goodness rocket engine. J-2X is coming together! In another month or so, it will be fully assembled and early this summer we will be demonstrating the first new, human-rated NASA rocket engine since 1975 (…yes, 1975, think: the fall of Saigon, Patty Hearst still on the lam, the Thrilla in Manilla – Ali v. Frazier III, Tiger Woods and Kate Winslet born, sentences being handed down for Watergate, the very first episode of Saturday Night Live, and me as the star kickball player during fifth grade recess…).

Two final notes for this article. First, I would like to thank Brian West for all of the engine assembly pictures and background information for these pictures. Keep up the great work! Second, I would like to thank my cat Kesey for his starring role in the center of the cat tree picture. Being that round and that lazy takes years of dedicated practice.

J-2X Extra: The Faces Behind J-2X, NASA MSFC, Part 2

Several weeks ago, the article here on the blog featured the an introduction to the upper management of the J-2X development effort. Now, I am going to introduce you to much of the next tier of our motley – yet talented – management crew, namely the subsystem managers here on the NASA side.

Rick Ballard is the systems engineering and integration manager. He is a product of the great Southwest U.S. and is therefore (it would seem to follow) a connoisseur and master chef of chili in multiple forms and variations, including some formulations can also be either eaten or used to scour barnacles from the hulls of ships. Rick is a graduate of Texas A&M who worked for support contractors, starting in 1987 first for Martin Marietta and then Sverdrup Technologies, before joining NASA over ten years ago. He is widely known for having an encyclopedic knowledge of space propulsion systems and is quite active in professional aerospace industry associations. When not working or spending time with his wife and two daughters, he can either be found participating in fencing or, as he puts it, “chilling” at his farm north of Gurley, AL.

Gary Genge, subsystem manager for Turbomachinery, like our chief engineer Eric Tepool, is also an Auburn University graduate and, like our chief engineer, began work at NASA in the turbomachinery branch. He started working for NASA in 1987. Back then, of course, the turbomachinery branch was immersed in Space Shuttle Main Engine (SSME) support. He was also the lead systems engineer for the Advanced Space Transportation Program and the NASA-side project manager for the Integrated Power Demonstrator (IPD) engine, which was a really cool technology demonstration engine using a full-flow staged-combustion cycle engine. Gary has three daughters and so, of course, has become an expert in attending dance recitals and gymnastics meets in his off hours. When not exercising this expertise, he has been known to golf, or play pool, or, once upon a time, far, far away from here, scuba dive.

The business office for the Upper Stage Engine Element office is led by Joan Presson, a native of North Alabama. While “business” is not technically a J-2X subsystem, without the flow, control, and accounting of the dollars and cents, nothing else can happen. The interesting thing about Joan is that, strictly speaking, this is her first gig as a “business manager.” It is not, however, her first time dealing with all that business stuff given that over her nearly 24 years with NASA she was manager or deputy manager for a variety of small projects in the space sciences area where business management was part of the role for the project manager. Joan has a Bachelor of Science in Engineering (major – electrical engineering), a Bachelor of Arts (major – English), and a Master of Administrative Science (concentration – project management). It was during her graduate work that she met her husband and today they have a 12-year-old boy. When not helping with his homework, or engaging in other activities with her son, Joan loves to get deep into hands-on house renovation projects.

Twenty years ago, Mike Shadoan started here at NASA MSFC straight out of the University of Kentucky graduate school. He’s still here, working hard, as always, but now diligently plugging away as our combustion devices manager. He started as a designer in the area of control systems and mechanisms and that’s where he earned the accolade of co-inventor of the year for MSFC for a selectively-locking knee brace design. For the last ten years, though, he’s worked all facets of combustion devices development. On the COBRA engine project, he was the subsystem manager for the thrust chamber assembly. On IPD, his job was geared more towards the manufacturing processes. On the Fastrac engine project, he was back focusing on design. In other words, he’s perfectly suited for his current job. But when not doing that, you can find him pursuing his hobby as a beekeeper or coaching either fast-pitch softball or basketball for any one of this three daughters.

The Upper Stage Engine Element office has set aside a management block dedicated to J-2X production. The person managing this block has the responsibility to establish what is necessary beyond the J-2X development effort in terms of supporting a flight program. Bill Jacobs, native of Rochester, New York and graduate of the University of Alabama, is the J-2X production manager. He began working here at NASA as a co-op in the mid-1980’s. His technical specialty back in those days was control system electronics. Working out of the engineering laboratory, Bill supported an alphabet soup of different projects and programs, from the Orbital Maneuvering Vehicle program to the National Launch System to the Fastrac engine development project. From there his career transitioned towards systems engineering and for quite some time Bill was stuck with the moniker of “Requirements Guru.” When not at work, Bill brews beer, plays tennis, and works on his photography. Those things are only possible, of course, when not spending time with wife and three daughters. Finally, Bill is one of those rare guys who played in his high school band 30 years ago and can still competently toot his trumpet when the mood strikes him.

A man with a diverse background within government employment is our manager for engine controls, valves, and instrumentation, Jeremy Richard. While he’s only been with NASA for about eight years, his total government service with Space and Missile Defense, the Army, and the Army Corps of Engineers comes in at 17 or 18 years (depending on how you add it up). Previously with NASA, Jeremy worked in the SSME office and even there sought a diverse experience spending a year and a half in California at the prime contractor site acting as a safety and mission assurance representative and also a year at the NASA Stennis Space Center in southern Mississippi in the SSME project resident office. He is a graduate of the University of Alabama in Huntsville, has four quite active children, and spends most of his off-hours time with his kids or working on his house and six acres of country land. An interesting additional fact about Jeremy is that he is a licensed preacher so should the need arise for an emergency, in-office wedding, we’re covered.

Our subsystem manager for engine systems hardware is Andy Hardin. If you’re wondering about what “gine system hardware” is, it’s basically everything not covered by the other hardware subsystems, which means that it consists of lots and lots of various piece parts. It’s probably appropriate then that Andy is our manager here given his diverse background. Andy spent several years in the Army, living in Louisiana and then three years in Fairbanks, Alaska. After the Army, he went back to school, earned his degree and pursued several jobs in the telecommunications field, working as engineering manager and program manager, including management of the establishment of a cellular network along the Gulf Coast. Andy has been working in support of NASA since 2003. He’s a golfer gifted with a broad spectrum of emotional display on the course, an excellent pool player, the world’s greatest collector of gadgets, and general household tinkerer/putterer. And, on top of that, he has two very young children. Busy man.

Like the business office, the Upper Stage Engine Element procurement office is not technically a J-2X subsystem. But considering that NASA as an agency buys products and services from the private sector to the tune of well over 80% of our budget, having competent procurement specialists in place is an absolute necessity. Luckily, we have Kim Adams leading our procurement office. Kim is an Auburn University graduate with a degree in management. She’s been with NASA for a total of 18 years and in her spare time dabbles in real estate. Trust me, if there was anyone that you’d want to take into a tough negotiation, it’s Kim. That’s not just because of her experience and intelligence, but because, as she says, she has a great poker face when needed. I’m sure that that has also been helpful in raising two sons, one of whom is a freshman at Auburn and the other is a tenth-grader and avid soccer player. When given the opportunity, Kim loves to travel, attends many Auburn football games, and is eagerly looking forward to a trip to Key West in May. And while she only keeps up today with some keyboard playing for fun, back in high school she played saxophone, xylophone, and piano.

Much earlier in this blog series, I provided a definition for “Datadog.” If, with that definition I’d wanted to include a photograph, I would have included an image of Marc Neely, our subsystem manager for assembly and test. Marc has spent the largest portion of his career, starting with NASA in 1985, supporting the various incarnations of the engine systems branch here at MSFC, the native home of those feral beasts known as Datadogs. In so doing, there are very few engine projects over the last two decades that Marc has not touched. Beyond that, he has interests in woodworking (he was once a carpenter) and art (once a subject of his collegiate studies as a younger man). Marc just recently got back from a trip to Nicaragua where his soon-to-be-doctor son got married to his soon-to-be-doctor and now daughter-in-law from that Central American country.

So, that’s just a further sampling of our office. These are my coworkers and friends. Beyond these folks, there are literally hundreds of people behind the J-2X development effort and they span the country. As we progress, I hope to tell you about more of the people involved.

J-2X Progress: Engine Assembly Starts!

Ready…
Set…
Go!!!

After so many requirements reviews and concept reviews and safety reviews and design reviews, and after so many trade studies and analyses and assessments, and after so much paperwork generation and processing, and after so many programmatic meetings and technical interchanges and integration exchanges and formal boards, after all that, we have finally begun assembling the very first J-2X, Engine 10001, at the NASA Stennis Space Center (SSC) in Building 9101.

In the end, truly, it all comes to this: the hardware. Everything that we do – and it is an astonishing amount of work – comes down to an operational piece of space launch hardware, a rocket engine (albeit a development unit first example). It is sometimes quite easy to forget that fact after being buried in the mountains of necessary details for several years. Yet that day has now arrived.

The Main Combustion Element (MCE), consisting of the Main Combustion Chamber (MCC) and the Main Injector (MI) mated together, arrived at NASA on 22 February 2011. While a number of piece parts and components have been arriving at SSC for weeks, it is the arrival of this sub-assembly that marks the start of assembly. It would not be too much of stretch to say that the rest of the engine, one way or another, hangs off the MCE. So you need that part to get the whole process started in earnest.

The drawing below shows the first steps in stacking the whole thing together.

Your first question should be, “What’s a Birdcage?” It’s actually a simulator for the nozzle. Because this is the first build of the engine and because the various components are not completing fabrication in the optimal sequence, we have found ways to expedite engine assembly such as the use of this nozzle simulator. The whole engine will be stacked and assembled with the Birdcage acting as the effective pedestal for the process. Then, when the nozzle does arrive, the assembled upper part of the engine will be lifted, the Birdcage will be removed, and then the assembled pieces will be lowered onto the actual nozzle assembly to be used for Engine 10001. Below is a photograph of the actual Birdcage sitting in its packing box at NASA SSC.

(Now, I’m not going to burst anyone’s bubble, but whoever first started calling this thing a “Birdcage” perhaps has a frightening impression of how large are the birds of southern Mississippi. Those are some awfully large holes.) Later, the Birdcage will be reused as the structural foundation for the assembly of the J-2X PowerPack Assembly to be tested next year.

The next picture is of the assembly area where the whole thing will be brought together. There is a raised floor on which the technicians will stand and there is a recessed area where the dolly will fit on which the engine is assembled. On the silvery metal carts shown – the so-called “bread carts” – the kits will be laid out for the next stage of assembly as the engine comes together.

One interesting little note about that picture of the assembly area is that in the upper left-hand corner you can see another engine within a big yellow piece of ground support equipment. That is an RS-68 engine that flies on the Delta IV vehicle. The J-2X assembly area sits right next to the RS-68 assembly area though they are distinctly separated.

Here are some other really cool pics:

Okay, so maybe “really cool” is a slight exaggeration. On the left is a corner of the kit staging area where, on a pallet in the back (can you see it?), sits the first pre-arranged kit of engine parts to arrive at NASA SSC. On the right are the two turbopumps for Engine 10001 still sitting in their shipping crates. Trust me, as the assembly process moves forward, the pictures will get better. Really.

So, J-2X is coming together. Over the next several weeks, I will be posting pictures and descriptions of the process. I do have to say, from a personal perspective, seeing this thing finally becoming a reality is quite gratifying. Thank you all for coming along and sharing the ride.